The Polyhedral Hypothesis
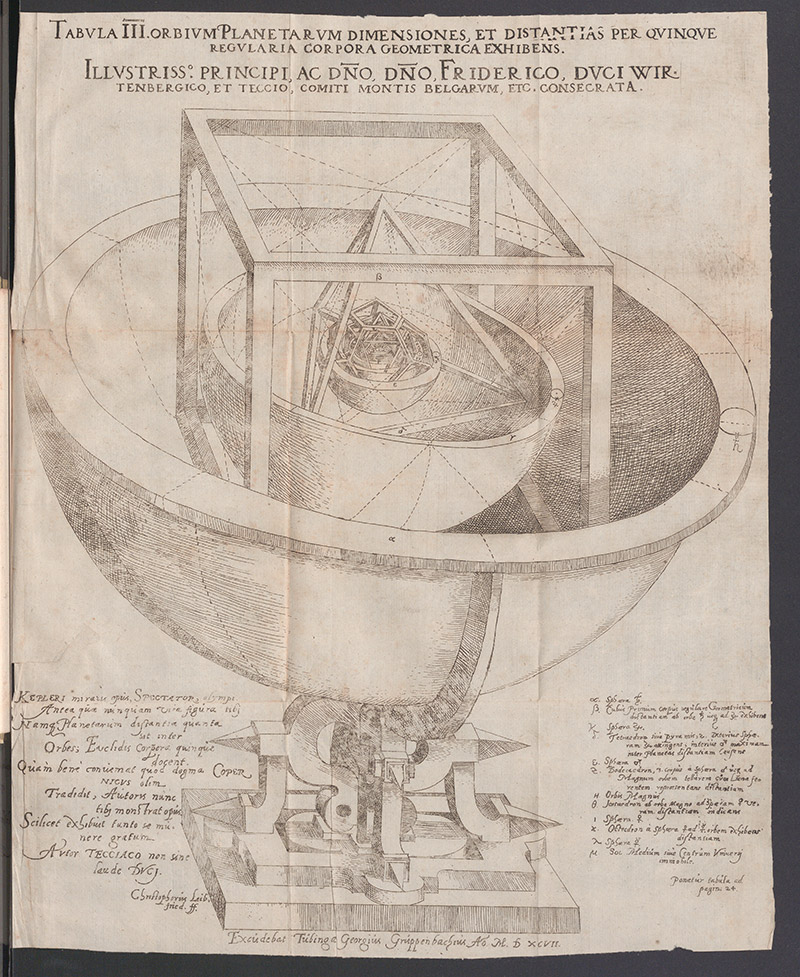
- Woodcut depicting a polyhedral model of the universe.
- Johannes Kepler (1571-1630).
- Prodromus dissertationum cosmographicarum, continens Mysterium Cosmographicum, de admirabili proportionem orbium coelestium, deque causis caelorum numeri, magnitudinis, motuumque periodicorum genuinis & propriis, demonstratum, per quinque regularia corpora geometrica.
- Tübingen: Georgius Gruppenbachius, 1596.
The most striking illustration of the Mysterium Cosmographicum is the woodcut depicting a polyhedral model of the universe. On July 19, 1595, while lecturing in Graz on the conjunctions of Jupiter and Saturn, Kepler realized that the ratio of the circle circumscribing an equilateral triangle to the circle inscribed in it was similar to the ratio of Saturn’s orbit to Jupiter’s orbit. This type of relationship inspired him to experiment with three-dimensional polyhedrons, concluding that the structure of the cosmos, or God’s geometrical plan, could be visualized by using five regular polyhedrons, also called the Platonic solids: tetrahedron, cube, octahedron, dodecahedron, and icosahedron. Kepler argued that each of the Platonic solids could be inscribed and circumscribed by a spherical orb. The nesting of these Platonic solids within one another, each of them being inscribed in a sphere, would generate six layers that are equivalent to the six known planets: Mercury, Venus, Earth, Mars, Jupiter, and Saturn. By placing the Platonic solids in the correct sequence (octahedron, icosahedron, dodecahedron, tetrahedron, and cube) Kepler realized that the spheres represented the sizes of each planet’s orbit around the Sun.